The Fraction Solution
∞ PART TEN ∞
PROBLEM CREATION EXAMINATIONS
 HOW FAR CAN AN OSOM STUDENT
HOW FAR CAN AN OSOM STUDENT
TRAVEL ON HIS OWN ROAD?
How far can an OSOM student travel on his own road?
Quite far.
Hopefully (and literally) to the stars.
It is my opinion that a student who is successfully grooved in to learning math the OSOM way should be able to take (and pass with flying colors) “Problem Creation Examinations.”
I suggest the following:
Along with the rest of his class, he takes all the usual examinations that accompany his textbook lessons. He must pass those textbook exams and be corrected on any errors he made on those tests. (Even if he scored 99%, he must still be thoroughly corrected on the 1% he missed – because that 1% of confusion at this level will compound later into greater and greater percents of errors both in school and in professional life.)
In addition, each semester (fall and spring), he takes two separate Problem Creation Exams, one in the middle of the semester and the other after the textbook’s final exam.
These special exams would consist of a list of the kinds of problems he was required to create, solve and check. What topics and how many? The kind and quantity of topics would come directly and only from his textbook and textbook exams he has just completed. (No surprises allowed. Step Two is firmly based on his textbook’s data which he has already mastered.)
Many math exams consist solely of numerical or word problems to be solved. Solving such problems is necessary and useful. But a more complete exam requires the student not only to solve problems but also to demonstrate real understanding of the terminology, axioms, theorems, concepts and purposes underlying the math of the lessons. Here is a question from Saxon Math, Algebra 2, Second Edition, Lesson 88, that requires the student to understand math principles and purposes:
“Write a short explanation of why [emphasis mine] the distance formula works, and include a discussion of why either point can be point I or point 2.”

To answer this question the student must actually think. He must understand why the distance formula works and express that understanding using his own words, math expressions and equations.
 A PROBLEM CREATION EXAM QUESTION
A PROBLEM CREATION EXAM QUESTION
To give you an idea of the rules, style and content of a Problem Creation Exam, here’s a single exam question covering division that might be used on a Problem Creation Exam for a 5th grade student.
These are not short, easy exams; they are comprehensive exams because they are given only twice a semester.
There is no time limit; the OSOM student may (or may not) spend some hours taking a Problem Creation Exam. But since the OSOM student has already mastered the topics covered in this exam, I expect they will be completed quite rapidly. The student who takes excessive time on these exams has raised a “red flag” (potential trouble.) This red flag should later be followed up by the examiner to discover if there are any confusions or uncertainties underlying the excessive time, regardless of his score on the exam – even a 100%.
Problem Creation Exams are not done in the student’s classroom. They are conducted and scored by one of the school’s independent OSOM examiner(s), an examiner specifically trained to conduct and score such OSOM exams properly. (Remember, there are no prepared answer sheets for created problems!)
These exams are done after the first roll call at the start of the school day on a Tuesday, Wednesday or Thursday. Prior to giving this exam, by direct questioning, make certain that the OSOM students are properly fed and rested and that they have no mandatory activities planned that would require them to leave the exam area or the school. Students are permitted to have bottles of water with them.
Needed formulas are stated on the exam so formula cards are not permitted.
If a particular student is taking a long time completing his exam, he may, with permission of the presiding examiner, take a break for food and a walk.
Background: By the end of 5th grade, the student has been studying and using division for about 2 years. In 4th or 5th grade, he learned to divide 2-digit divisors into 4- and 5-digit dividends. He understands thoroughly the interrelationship and interdependence of multiplication and division. He has already passed all his textbook’s regular math exams, including exams that include division problems with 2-digit divisors.
Since Problem Creation Exams are done only twice a semester (which equals four Problem Creation Exams during a 10-month school year), this exam would probably include 15-25 questions, each covering one major topic he has mastered in the period preceding this exam. I might (but would not normally) use questions that are based on minor topics.
The following is ONE question with many individual parts. Note that I have intentionally included everything – definitions of important terms, purposes of the topic, sketching of mathematical principles, and application essays into this one question (along with the expected problem creation, problem solving and checking answers steps) to demonstrate what an OSOM student should know about his math. Most exam questions would be much shorter — or else the exams would never get completed!
Note: Pencils and paper are provided by the school.
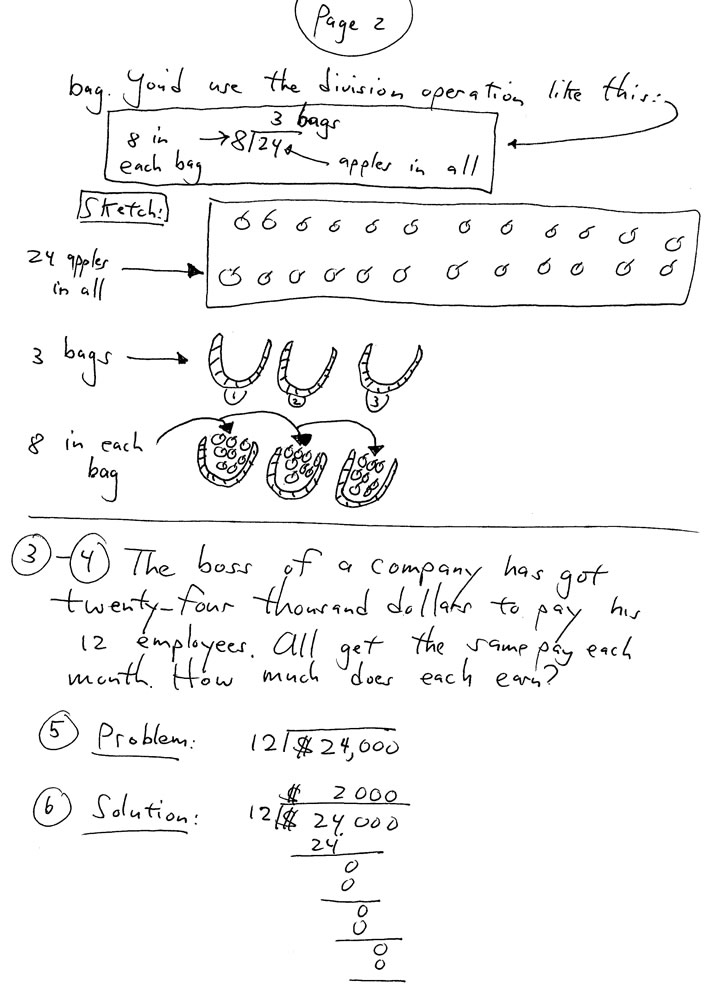
- Define the words “division”, “dividend” “divisor” and “quotient.”
- Describe in writing the purpose of division. Sketch that purpose.
- Now make up (invent, create) a division word problem that involves dividing a specific quantity of money. Your problem must involve a 5-digit dividend and a 2-digit divisor. You must choose which factor in your problem is the missing factor, and your problem must demonstrate that you know the difference between the two factors involved in your division problem.
- Express the entire problem in written words, including the numbers (use no numerals in your word problem.)
- Write down the problem you have just created using standard notation numerals and division symbols.
- Then, solve your created problem using only your book’s solution procedure. No mental math is allowed. Do not use a calculator. Every calculation is done on paper. Each step must be written down clearly. (Leaving out critical solution steps will be considered an error.)
- Next, check your answer. Again, use only your book’s checking procedure. No mental math is allowed. Do not use a calculator. Every calculation is done on paper. Each step must be written down clearly.
- After your division problem is created, solved and checked, write an essay describing specifically and exactly how you can use the above division procedure in your life outside of school. Your essay must include at least two specific (not general), real-life applications of the above division problem: 8a) The first specific application involves how you could use the above division of money now, as a young person. 8b) The second specific application would involve how you might use the division of money as an adult.
“You can use real-life situations that you or other people you know have been involved in. You can also make up (imagine, invent) specific situations or experiences that could actually occur in real-life circumstances.”


 PROBLEM CREATION EXAMINATION AWARDS
PROBLEM CREATION EXAMINATION AWARDS
Although “Problem Creation Examinations” now exist only as an idea, it seems reasonable to me that such a study/learning activity would be highly beneficial, raising the student’s overall learning of that semester’s math to a new height.
Students who partake in a program of Problem Creation Examinations should, I believe, receive a special award each semester acknowledging superior accomplishment and competence. Note that if a student was in an honors’ math class, this OSOM award would be given as a higher accomplishment than simply completing the honor’s class. Naturally, such an award would be noted in their transcripts, the official papers documenting their educational work, and would accompany college and employment applications.
Eventually, as universities and employers come to understand the extra value that such students bring to their studies and work, such an award would become highly sought after.

