Bruce Silton
presents
The MathArt Series
∞ Works of Art ∞
from the World of Mathematics
Series Two: Educational MathArt

Ten New Digits
 Educational MathArt
Educational MathArt
“Educational MathArt” has the purpose of communicating the ideas and language of math as a subject to be studied, learned and used. But, since it is also art, I try to do this in a way that is not only useful and practical but visually interesting . I speak with personal truth when I tell you that mathematics has an aesthetic quality — the quality of beauty. I try to convey that aesthetic in my Educational MathArt drawings as well as in my Creative MathArt.
Section One:
Whole Numbers and Fractions
By 2008 (after 7 years of full-time tutoring), I had accumulated a lot of useful knowledge about how to successfully educate young people in the basics of arithmetic. I decided to write down the core of my knowledge for others to use. The result was a book (unpublished) called The Key Words of Basic Math. The book’s 90 + illustrations were done in pencil on white paper. My original intention was to upgrade the pencil to ink but….
Most of the text and illustrations from The Key Words of Basic Math will appear in MathWords (another page on www.MathCreativity.com). A few of the pencil drawings from Chapter 13, “Fractions”, are reproduced here (without the text) as MathArt.
These illustrations reflect the way I introduce the fundamentals of fractions to young students. I start with whole numbers, explain them carefully, and only then introduce the math fraction. My students never get lost.
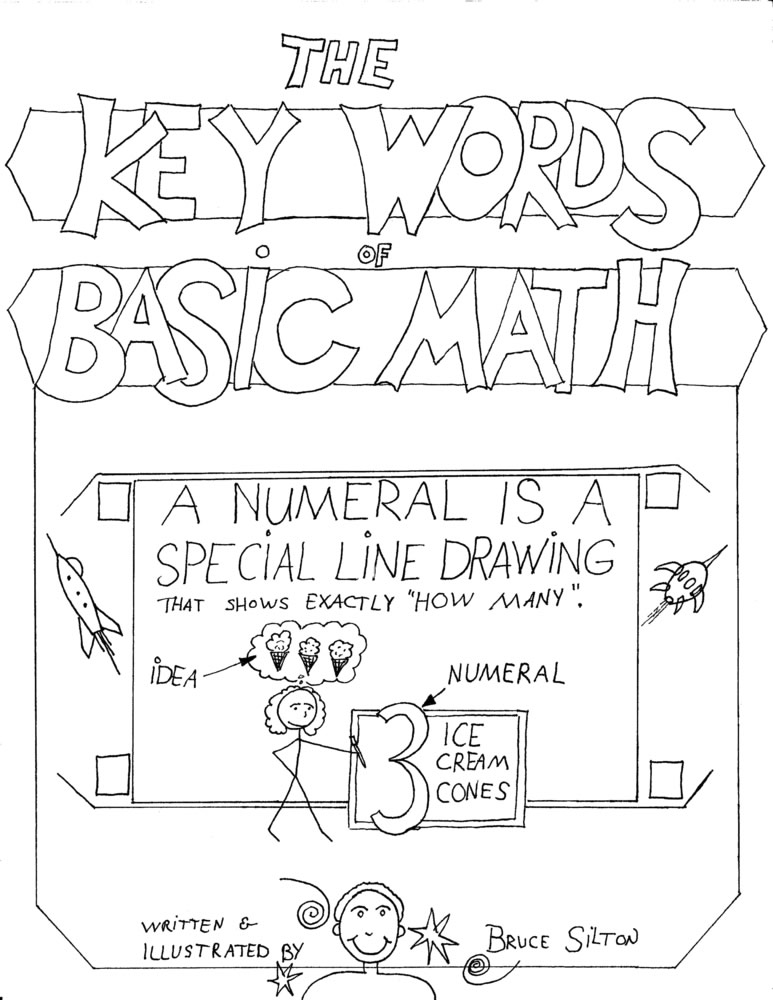
Design for the Book Cover
Whole Numbers Represent Whole Things

Two Whole Squares
When to Use Whole Numbers
 Whole Groups Need Whole Numbers
Whole Groups Need Whole Numbers
 Whole Numbers: One Chocolate and One Box of Chocolates
Whole Numbers: One Chocolate and One Box of Chocolates

Equal Parts of Whole Things are Represented by Fractions

A Fraction Is a Number
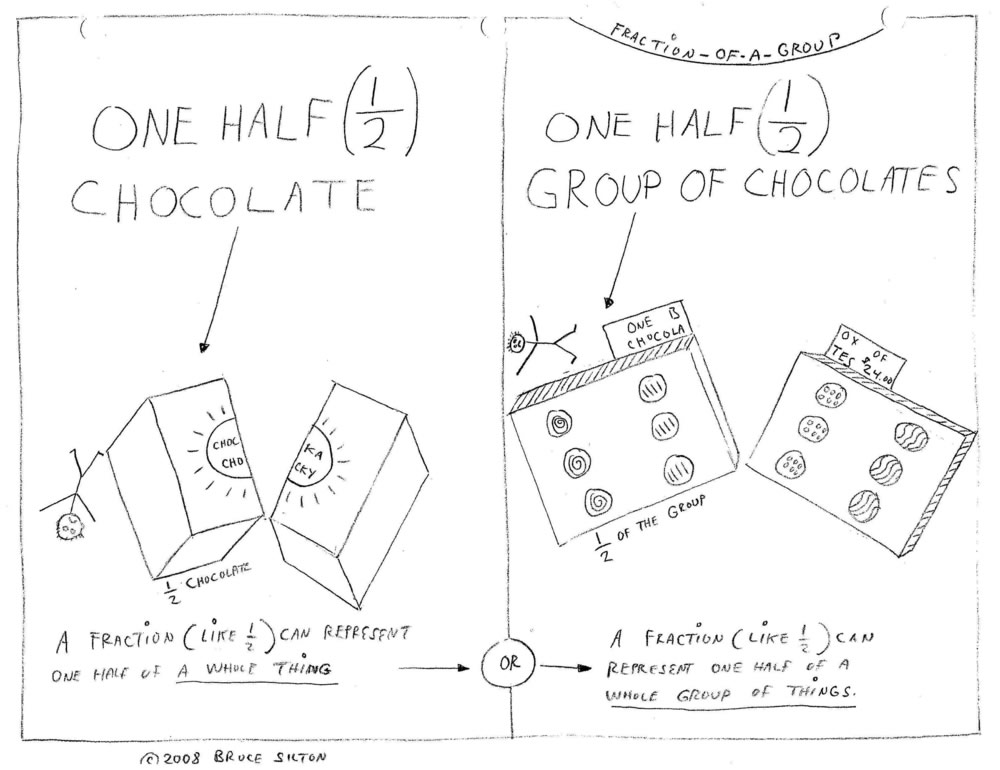 One-Half
One-Half
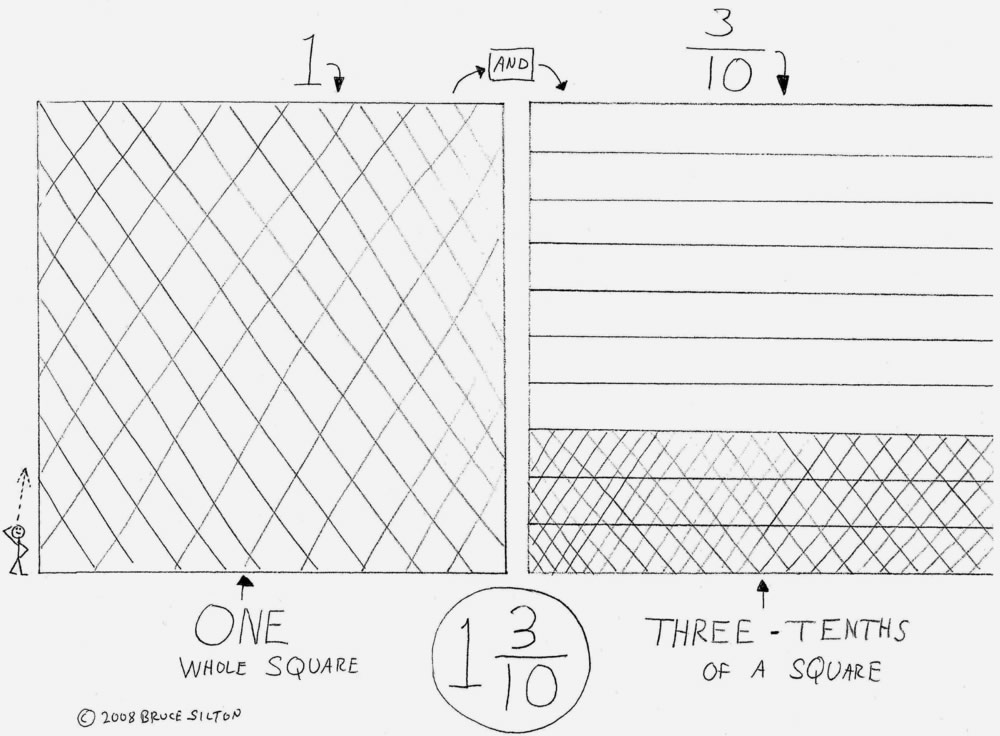
A Whole Number and a Fraction

White MathArt on Black Drawing Paper
A few years ago, soon after New Years Day, my sister Leslie’s Christmas gift arrived – a set of colored drawing pens using gel inks. The pens were accompanied by a pad of 9 inch x 12 inch heavyweight, black drawing paper, suitable for gel pens. After testing a few pens on the black paper, I settled on the white pen.
I trimmed 14 sheets of the 9 x 12 paper to 8 1/2 by 11 (so I could make copies on my home printer) and began to work. Over the next few weeks, writing and designing evenings and weekends, I produced the eleven works of White MathArt on Black Drawing Paper that appear in this website page.
 Section Two:
Section Two:
Alternative Arithmetic Algorithms
Definition of Algorithms: In the subject of math, an algorithm is specific method for solving a certain kind of math problem.
Prior to receiving Leslie’s gift, I had completed a project to learn some alternative methods of adding, subtracting, multiplying, and dividing — methods different from those taught in most America schools. After 68 years of doing basic arithmetic the same way, this was a liberating experience.
After a student has mastered his textbook’s usual algorithms for arithmetic, learning alternative methods frees up the student’s mind and increases his ability to use math more effectively. (Another paper on this website –“The Other Side of Math” – covers this procedure in detail.)
These first four designs show alternatives for the four basic operations of arithmetic.The border designs incorporate digits and numerals from older numbering systems, including Babylonian, Mayan, and Egyptian symbols.
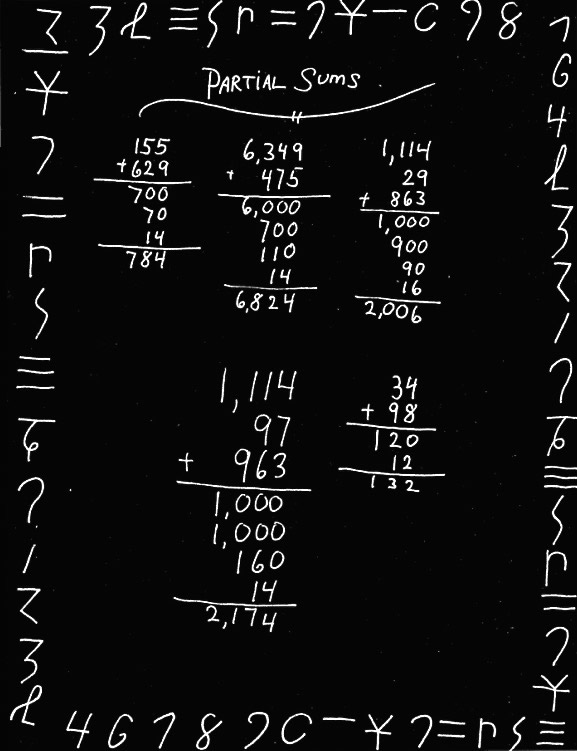
Alternative Algorithms: Partial Sums
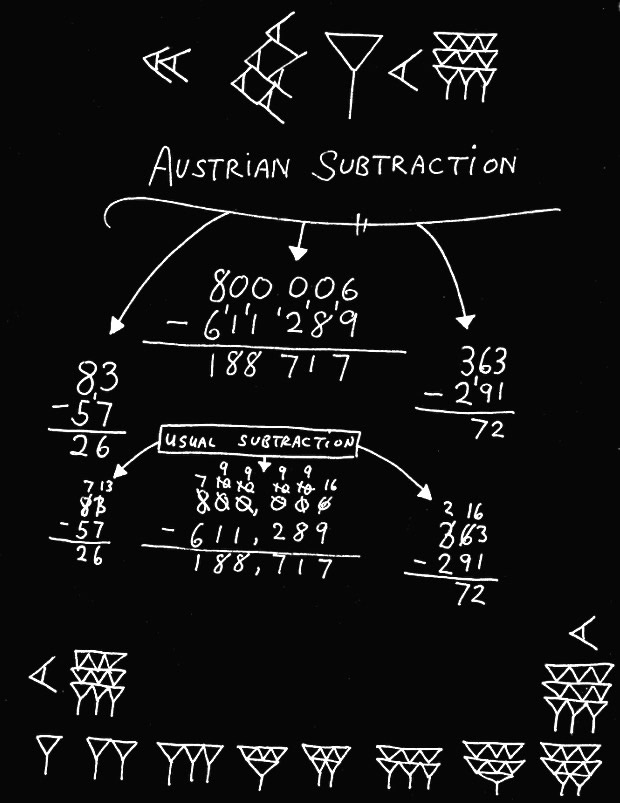
Alternative Algorithms: Austrian Subtraction
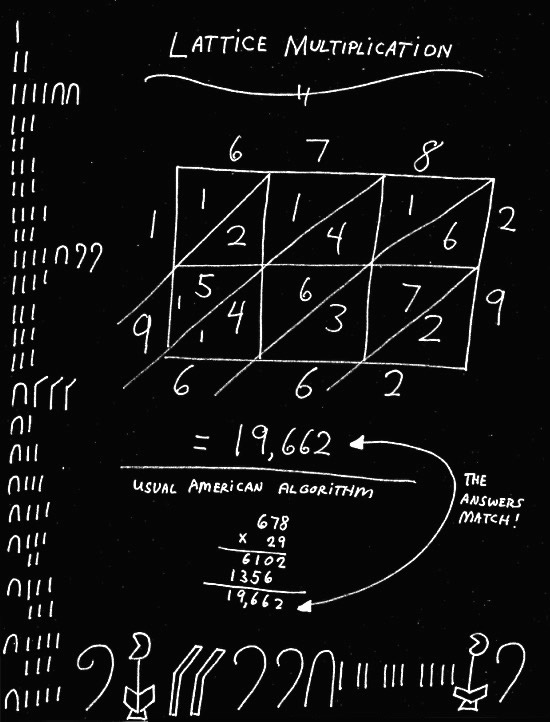
Alternative Algorithm: Lattice Multiplication (13th Century, A.D.)
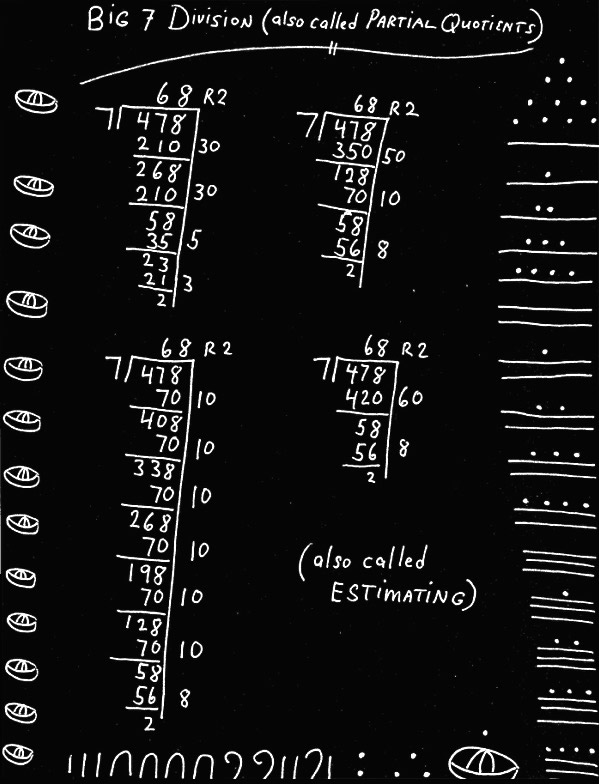
Alternative Algorithm: “Big 7” Division
 Section Three:
Section Three:
AlgebraArt
In 2010, facing the sad truth that my personal knowledge of high school algebra was ancient history (from1958 or 1959), I began a math adventure: a cover-to-cover study of every page, every lesson, every problem, and every exam of John Saxon’s Algebra One (Second Edition) – without the help of a teacher.
Four years later, when I had completed all 132 lessons (with a 100% final exam!), it was time to communicate some of the pleasure, excitement and pure intellectual happiness I had experienced while navigating my way through a modern (and excellent) algebra text.
The following seven designs incorporate both the actual algebra plus border designs from ancient numbering systems.
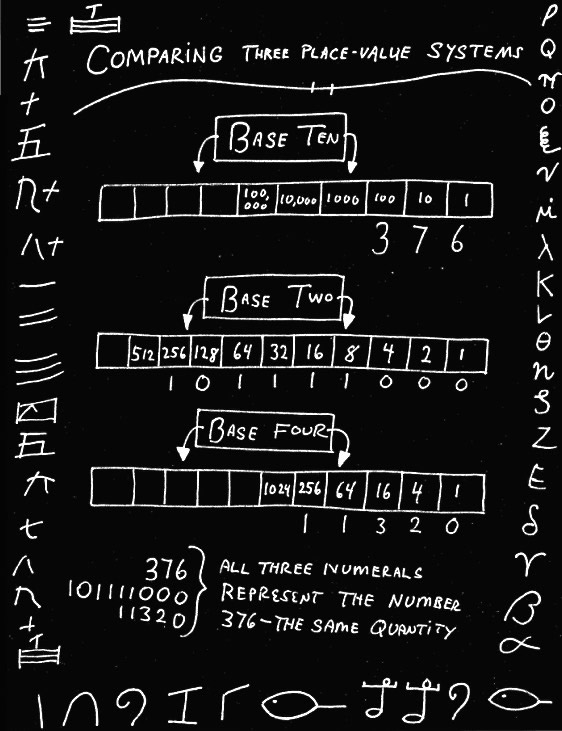
Comparing Place-Value Systems:
Base 2, Base 4 and Base 10
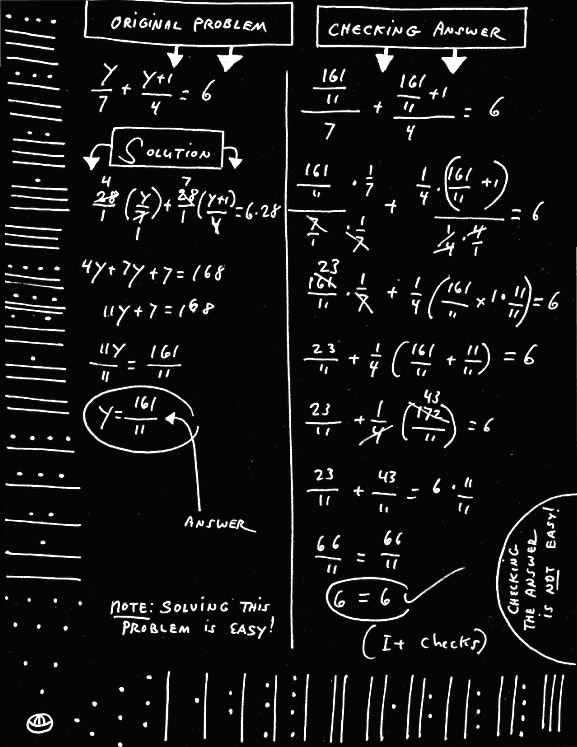
Using the Lowest Common Denominator
to Eliminate the Denominator of a Rational Equation:
“Solving is Easy, Checking can be Hard”

Division of a Four-Term Polynomial by a Binomial
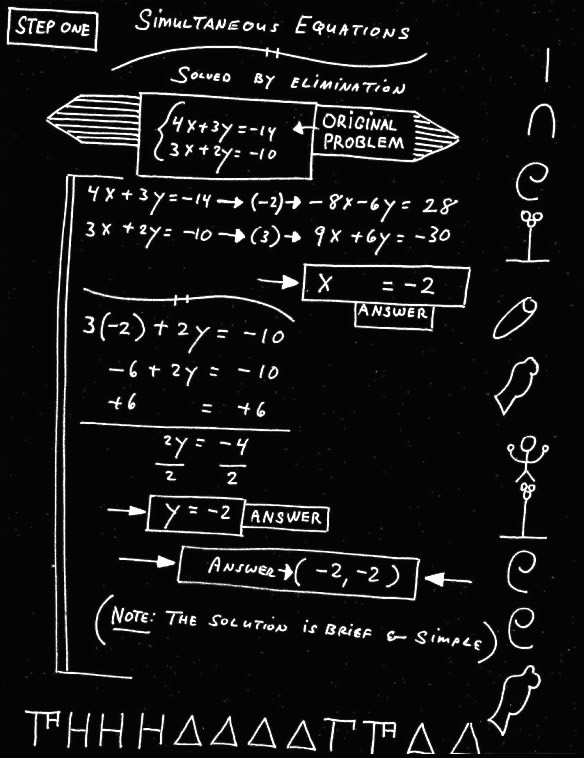
Solving a System of Two Simultaneous Equations by Elimination
Solution: (-2,-2)
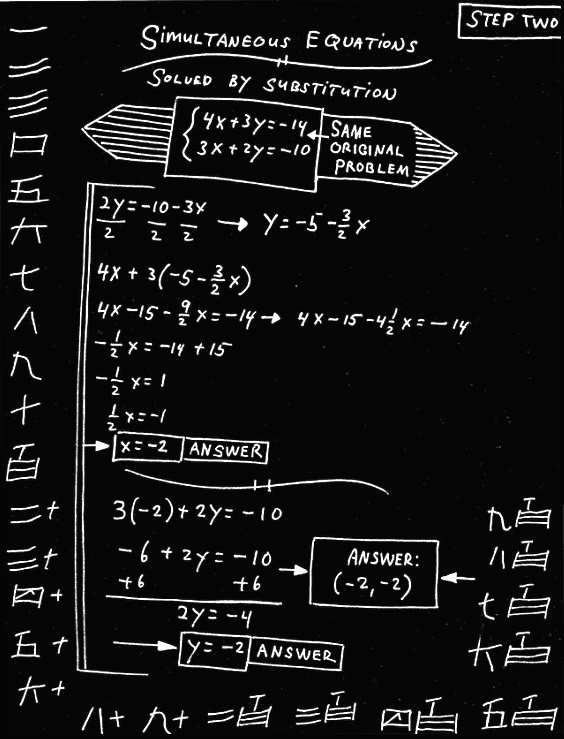
Solving the Same System of Two Simultaneous Equations by Substitution
Solution: (-2,-2)
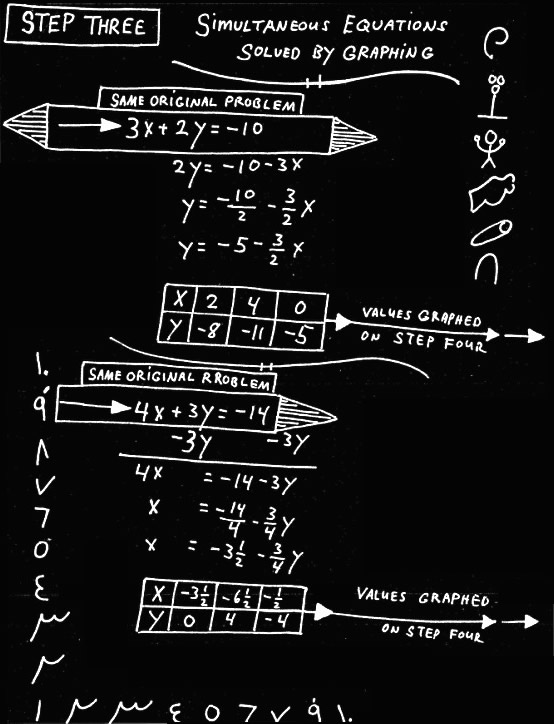
Using Tables to Find Values for x and y
Prior to Graphing the Same System of Two Simultaneous Equations
 Graphing the Same System of Two Simultaneous Equations
Graphing the Same System of Two Simultaneous Equations
Solution: (-2,-2)
(They all check!)
The end
Copyright © 2016, 2017 Bruce Silton
 A Note from the Artist
A Note from the Artist

Do you also create your own MathArt?
If you do AND if would like to share your MathArt with me, let me know.
But if you don’t create your own MathArt, would you like to start?
You can contact me using the contact page on this website (www.MathCreativity.com) or by using the contact page on my artist’s and designer’s website( www.BruceSilton.com)
 About the Artist
About the Artist

In the Swiss Alps
(Photograph by Mia Silton)
Bruce Silton is a tutor at Clearwater Academy International, a preschool –12th grade private school in Clearwater, Florida. Since March of 2000, he has delivered approximately 17,000 tutoring sessions to students of all ages. Some 10,000 of those tutoring sessions have been devoted to helping students overcome difficulties learning math. While working with students to resolve their math difficulties, he become interested in the visual aesthetics of mathematics and began creating his MathArt.
His fine art and design can be viewed at his artist’s website: www.Bruce Silton.com
In and Out of the Math Hole